Introduction
The phase rule is a fundamental concept in physical chemistry that helps us understand the nature of matter and the changes it undergoes. The concept was first introduced by the American chemist J. Willard Gibbs in 1875. The phase rule is a simple equation that relates the number of phases, the number of components, and the number of degrees of freedom in a system. In this blog post, I will introduce you to the phase rule, its definition, and how it is derived.
The phase rule defines the maximum number of independent variables that can be varied in a system at equilibrium without changing the number of phases. The variables that can be varied include temperature, pressure, and the composition of the system. The phase rule is an essential tool for understanding the behavior of materials, including liquids, solids, and gases.
Phase rule:
The Gibbs’ Phase Rule, based on thermodynamics, provides the theoretical framework for describing the chemical state of a (geologic) system and predicting the equilibrium relations of the phases (minerals, melts, liquids, and vapors) present as a function of physical conditions like pressure and temperature. We may also create phase diagrams using Gibbs’ Phase Rule in order to represent and understand phase equilibria in heterogeneous geologic systems.
Phase rule relates the various changing elements or variables of a system in a thermodynamic equilibrium. A system is said to be in thermodynamic equilibrium when it is separated or isolated from its surrounding environment. The separation can be obtained by considering a closed container as a system. According to the number of distinct phases and chemical components in the system, the phase rule describes the potential number of degrees of freedom in an enclosed system at equilibrium. J.W. Gibbs came to this conclusion in the 1870s. The phase rule is now often referred to as the Gibbs phase rule throughout the entire world. We shall talk about how the phase rule was derived in this essay.
The total number of independently varying intense variables—the most thermodynamic characteristics, like temperature or pressure, that may be changed simultaneously and arbitrarily without affecting one another—is known as the number of degrees of freedom. One pure chemical system serves as an illustration of a one-component system, while two-component systems, like mixes of water and ethanol, have two chemically distinct components. Solids, liquids, and gasses are common phases.
The variables in a system may vary from one place to another depending upon the type of system. The various variables in a phase rule are the number of phases (P) whether the phase is solid, liquid or gas or a combination of any three forms of matter, chemical composition (number of chemical components) C whether pure compounds or elements and the number of degrees of freedom F and includes temperature, pressure and composition in percent. The phase rule can be represented as:
Where, F is the degrees of freedom
C is the number of chemical components and
P is the number of phases
For 2 phase systems and with 2 components, the number of degrees of freedom is two, and in such cases any value of temperature and pressure can be attained within the prescribed limits.
In the absence of gravitational forces and electromagnetic forces, the equilibrium state of a heterogeneous system is not affected, the above rule is known as Gibbs Phase rule. It states, if the equilibrium in a heterogeneous system is not affected by gravity or electromagnetic forces, the number of degrees of freedom is given by:
This equation gives a mathematical relationship and helps in determining the stability of the various phases present in the system at equilibrium.
The rule’s underlying tenet is that the intense variables are constrained by phase-to-phase equilibrium. More precisely, the chemical potentials of the phases must be equal since they are in thermodynamic equilibrium with one another. The amount of degrees of freedom is determined by the quantity of equality relationships. For instance, if the chemical potentials of a liquid and its vapor are dependent on temperature (T) and pressure (p), then each of those variables will also be dependent on the other due to the equality of chemical potentials.The rule is valid as long as only temperature, pressure, and concentration affect the equilibrium between phases and not gravitational, electrical, magnetic, or surface area forces.
If some components in a multi-component system are in chemical equilibrium with one another, then the number of components that need to be enumerated may be less than the overall number. One component would be a monomer (simple molecule) in equilibrium with its dimer (two molecules chemically linked together).
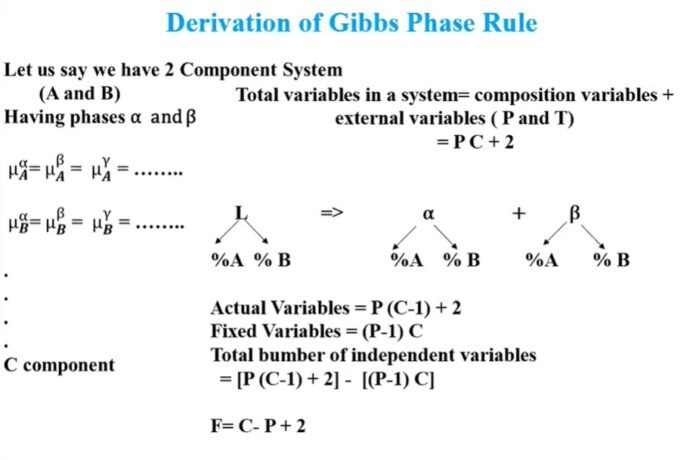
Derivation of phase rule
The phase can be easily understood and derived by using the Gibbs-Duhem equation, which forms the basis of thermodynamics. It gives a clear understanding of the relationship between the various variables in a thermodynamic system at equilibrium. The Gibbs-Duhem equation clarifies the relationship between pressure (P), temperature (T) and potential for chemical components (μ). This equation is as follows –
The simple way to obtain the equation of the phase rule is to understand the components in each phase and is given by [P (C-1). Thus, the total number of variables is equal to
P (C-1) + 2 equation i
The total Number of equilibrium for each component’s each phase is P-1
Now for C number of components, the number of equilibrium = P (C-1)
Therefore, total number of equilibria
E = C (P-1) equation ii
After analyzing the equation 1 and 2, we get the following results –
This is the required equation of Gibbs Phase rule.
Thus the three variables are present in the phase rule equation, these are:
1. Phase (P): Any substance that can be isolated physically from a system is said to be a phase. The phase shows the form of a material present in the system whether it is in solid form, liquid form or in a vapor state. A phase can also be a mixture of two or more components or elements having both physical as well as chemical characteristics which they share with each other. According to the definition of a phase, it is “an homogenous, physically distinct, and mechanically separable piece of system, which is separated from other similar portions of the system by definable boundary surfaces.”
2. Chemical composition (C): It shows the total number of components that can be present in a system at a particular instant of time.The minimum number of independently variable constituents that allow the composition of each phase to be represented in the form of a chemical equation is referred to as a component.
3. Degrees of Freedom (F): The degree of freedom signifies the number of variables that can be changed in a system without having any effect on the state of the system i.e., the state should remain the same before and after the variable has been changed. The variables may be temperature, pressure etc. The bare minimum of independent variable elements, such as temperature, pressure, and phase concentration, that must be set in order to fully define a system’s condition is referred to as its degree of freedom.
Quintuple and sixtuple points have been observed in colloidal mixtures, defying the Gibbs phase rule. However, it is argued that in these systems, the rule can be generalized towhere M, which takes into account additional parameters of component interaction, such as the diameter of one type of particle in relation to the diameter of the other particles in the solution.
Limitations of Phase rule
- It can only be used on systems that are in equilibrium. As a result, it is not very useful for systems that reach equilibrium slowly.
- It only relates to one equilibrium system and gives no information about any additional potential equilibria in the system.
- Because it only takes into account the number of phases, not their amounts, it necessitates the greatest amount of caution when determining the number of phases present in an equilibrium condition. Hence, even the smallest amount of phase contributes to the overall number of phases.
- It stipulates that all system phases must exist concurrently and be subjected to the same pressure and temperature conditions.
- The phase rule is a powerful tool for understanding the behavior of materials. However, the phase rule has some limitations. The phase rule assumes that the system is in thermodynamic equilibrium. In practice, it is difficult to achieve thermodynamic equilibrium in real systems. The phase rule also assumes that the components in the system mix ideally. In practice, many systems do not mix ideally, and the phase behavior may be more complex.
- Another limitation of the phase rule is that it does not take into account the kinetics of the system. The phase rule tells us about the thermodynamic equilibrium of a system but does not tell us how long it will take for the system to reach equilibrium. In some cases, the kinetics of a system may be very slow, and the system may not reach equilibrium in a reasonable amount of time.
The phase rule is a fundamental concept in physical chemistry that helps us understand the behavior of materials. The phase rule relates the number of phases, the number of components, and the number of degrees of freedom in a system. The phase rule is derived from the fundamental principles of thermodynamics and the Gibbs-Duhem equation. However, the phase rule has some limitations, including the assumption of thermodynamic equilibrium, ideal mixing, and neglect of kinetics.
Recommended Articles:
Derivation Of Lens Maker Formula: Assumptions, Derivation, Applications, And Limitations
Derivation Of Lorentz Transformation: Introduction, Formula, Transformation, And significance
Derivation of Mirror Formula: Terms, Formula, Assumptions, And Derivation
Derivation of the One-Dimensional Wave Equation
Derivation Of Orbital Velocity: Introduction, Derivation, Escape, Relation And Factor
At triple point, all the three phases of a system exist and during this point degree of freedom is zero which means that the invariant reaction takes place at this point. At triple point, the value of P is three meaning all the three phases are present in the system at thermodynamic equilibrium. Triple point can also be defined as the temperature and pressure at which all the three states or phases whether solid, liquid or gaseous state coexist at equilibrium. All three phases exist in a water system at triple point because the water system forms a single component system. At the triple point in the water system, C=1, F=0 and we get the value of P=3 using the Gibbs Phase rule equation. It is the lowest temperature in a system at thermodynamic equilibrium at which the Liquid phase is in a stable state at constant or given pressure. It is a uniform or homogenous, concrete mixture of a group of substances that melts or solidifies when temperature falls below the melting point of any of the ingredients present in the mixture. In other words, eutectic point is the temperature and pressure at which a mixture in liquid state changes to the 2 solid phase at a particular instant of time when the temperature is reduced or upon cooling. Derivation of Phase rule FAQs
What is a triple point?
What is the number of phases at triple point in a water system?
What is the Eutectic point?