Derivation of Kinetic Energy

As we know that energy is neither created nor destroyed but changes its form. Energy or work that is involved by the body while moving is termed by the name Kinetic Energy.

Introduction

Understanding kinetic energy is essential to understand how moving objects behave. It is essential for the investigation of collisions and the movement of particles in fluids, for instance. The work-energy theorem, which connects the work done on an object to the change in its kinetic energy, forms the foundation for the formulation of the kinetic energy formula. As a result, studying mechanics, thermodynamics, and fluid dynamics, among other branches of physics, requires a thorough grasp of kinetic energy.

The energy that an object possesses as a result of its motion is known as kinetic energy, which is a fundamental notion in physics. A body at rest, that is it has got zero velocity, therefore it has zero kinetic energy . It is a scalar quantity that is equal to one-half of the product of an object’s mass and its square velocity. In other words, an object’s kinetic energy is directly proportional with both its mass and the square of its velocity. The unit of measurement for kinetic energy is the joule (J), which is equivalent to . Before deriving kinetic energy, we must know the notion of work and Kinetic energy famous by the theorem that is Work energy-Theorem.

Work energy-Theorem

The work-energy theorem is a fundamental principle in physics that relates the work done on an object to the change in its kinetic energy. The theorem states that the net work done on an object is equal to its change in kinetic energy, which is the energy it possesses due to its motion. In other words, work is a form of energy, and the total work done on an object is equal to the change in its kinetic energy. To derive work energy relation, we start with equation of motion with acceleration , initial and final velocity and given as-

————–(1)

Multiplying equation (1) by on both side, where m is mass of body, we get

—————(2)

We can generalize above equation in three dimension as dot product between acceleration a and displacement s as

—————-(3)

The left side of the equation (3) is the difference in the quantity ‘half the mass times the square of the speed’ from its initial value to its final value. We call each of these quantities the ‘kinetic energy’, denoted by . The right side is a product of the displacement and the component of the force along the displacement. This quantity is called ‘work’ done by force along the displacement and is denoted by . Now Equation (3) can be written as-

———————–(4)

Where, and are the initial and final kinetic energy of an object. Equation (4) shows The change in kinetic energy of a particle is equal to the work done on it by the net force which is Work- energy Theorem.

Derivation of Kinetic Energy

We can derive kinetic energy in two ways, one by algebra method and another by calculus method. So let’s derive a formula for kinetic energy using the algebra method and then we will also derive them by using the calculus method. Starting with work energy theorem and using newton’s second law of motion, we can write as follows-

—————(5)

Using equation of motion and rearranging , equation (5) can be written as-

Or

Substituting in equation (5), we get-

,

When the body is at rest, initial velocity is zero, the only energy a body acquires white moving with velocity v, is given as-

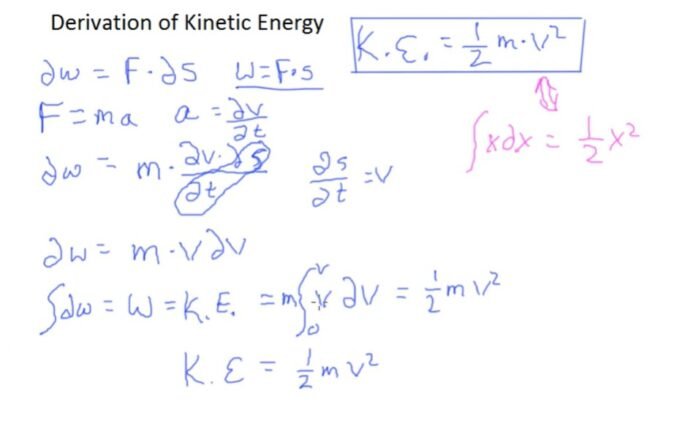
Another, method of deriving expression of kinetic energy is by calculus method as discussed below-

Again starting with work energy theorem and from newton’s second law of motion, we have-

Where, , on integrating both sides of equation in the limit from initial velocity to final velocity

When initial veocity is zero then,

Recommended Articles:

Derivation Of Drift Velocity
Derivation of Equation of Motion: Introduction, Methods, And Equations
Derivation of Escape Velocity: Introduction, Formula, And Earth
Hall Effect: Introduction, Theory, Principle, Principle, And Applications
Heat Equation: Introduction, Assumptions, Derivation, And Applications