Angular Momentum and Its Conservation, Applications, and Examples

Introduction

Angular momentum is a concept in physics that describes the rotation of an object around its axis. It is a crucial concept in mechanics and has applications in many fields, such as astronomy, engineering, and sports. We will find out what angular momentum is, how it works, and its examples.

What is angular momentum?

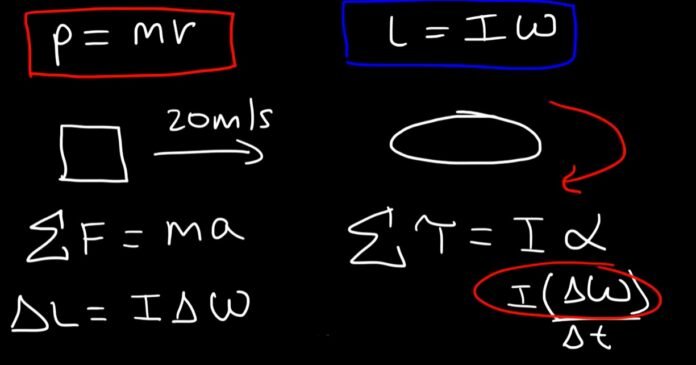
Angular momentum is a measure of the rotational motion of an object. It is defined as the product of the moment of inertia and the angular velocity of the object. In simpler terms, it is the product of the mass of an object, the distance of the mass from the axis of rotation, and the speed at which the object is spinning. The equation for angular momentum is as follows:
L = Iω

where L is the angular momentum, I is the moment of inertia, and ω is the angular velocity.

Angular momentum is a vector quantity, which means it has both magnitude and direction. The direction of angular momentum is perpendicular to the plane of rotation, and its magnitude is directly proportional to the speed of rotation and the distance from the axis of rotation.

Conservation of Angular Momentum

Angular momentum is conserved, which means that it remains constant unless an external torque is applied. This concept is known as the conservation of angular momentum. When no external torque is applied to a system, the total angular momentum of the system remains constant.

The conservation of angular momentum is a consequence of Newton’s third law of motion, which states that for every action, there is an equal and opposite reaction. In a closed system, the total angular momentum remains constant because any change in one part of the system is balanced by an equal and opposite change in another part of the system.

Applications of Angular Momentum

The conservation of angular momentum has important implications in many areas of physics. It explains why ice skaters can spin faster by pulling in their arms and why satellites stay in orbit around a planet without losing altitude.

1. In astronomy, angular momentum plays a vital role in the formation of planetary systems. As gas and dust particles in space collide and coalesce, they form larger and larger bodies. These bodies then start rotating, and their angular momentum increases. Eventually, they become planets or other celestial bodies.
2. In engineering, angular momentum is used in the design of rotating machinery, such as turbines and motors. These machines use the principles of angular momentum to generate power or produce movement.
3. In sports, angular momentum is a key factor in many activities, such as figure skating, diving, and gymnastics. Athletes use their bodies’ angular momentum to perform spins and flips, and they can manipulate their momentum by changing their body position or speed of rotation.

Examples of Angular Momentum

To understand angular momentum better, let’s look at some examples:

1. A figure skater starts spinning with her arms extended, and then she pulls them in close to her body. As she does so, her angular velocity increases, and her moment of inertia decreases. However, her angular momentum remains constant, as there is no external torque acting on her. By decreasing her moment of inertia, she can spin faster without using any external force.
2. A planet orbiting around a star has angular momentum. As it moves through space, it rotates around its axis, and its angular velocity remains constant. However, the distance between the planet and the star changes, so the planet’s moment of inertia changes. Despite these changes, the planet’s angular momentum remains constant.

Recommended Articles:

What is the Angular Momentum of an electron
Angular Momentum About Fixed Axis
Angular Displacement: Introduction, Motion, and Formula
Angular Acceleration – Formula, Definition, Application, and Factors
The Angle of Incidence